Triangular Francisco

also known as: TF, TFM, TriFran

I basically invented this method on a whim. I was talking in the #rubik chat (which I used to regularly attend) when Kirjava (Thom Barlow) posted an omegle conversastion he'd had with a stranger. The stranger said he could solve the Rubik's Cube, and furthermore said that he used the "triangular francisco method" and averaged something like 5 seconds (probably false). I thought that was a pretty cool name for a method, and after some thinking I came up with a reasonably fast method based on the idea of a triangle. I wrote the method up on the SpeedSolving forum less than 20 minutes after the conversation got posted.

As it turns out this is actually a decent method; I don't know all of the algs (particularly for the CLS step), but I've achieved an average of 100 of under 20 seconds. It probably won't get you world-class speed, but it's kinda fun and pretty unique.

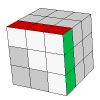
This step can also be called the B2 Bomber, after a certain Go pattern. The goal is to solve five pieces around the center to form a triangular formation. This can be fully planned during inspection; it's a little harder to do than a cross, but still not too bad. Personally I like to first plan a 2x2x1 block and then add on two corners; you could also combine a 1x1x3 block and a corner-edge pair. There are 4 possible triangles on each color (so, 24 if you're color neutral) and thus plenty of possibilities to check.

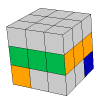
Place the triangle on the bottom, with the unsolved parts in the front (I generally put the unsolved corner at front-right). Now the goal is to insert the four edges of the middle layer. There are a bunch of efficient ways to do this. For instance, if the edge is in the top-front position and you want to bring it into the front-right slot, you can use R U' R' or r U r', depending on the edge's orientation. If the edge is in bottom-front, you can use r U' r' or r U2 r' R U' R', again depending on its orientation. Note that all of these moves can also be done on the left, by simply moving the unsolved corner on the bottom to the front-left position. This is useful if an edge gets stuck on that side. When you're done with an edge and you want to do another one, I find it's best to do a u or u' move (which is performed basically the same way as a U or U').

There are some additional tricks you can use here too. For instance, R2 E R2 and R2 E' R2 are useful for some situations where an edge is stuck in the wrong place. You can also do moves like R u R'. There's actually a lot of freedom in this step, so it's worth it to play around and see what you can do.

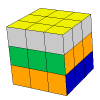
ESO stands for "edge slot + orient". In this step, the goal is to insert both bottom layer edges, while also orienting the top layer edges. There are several ways to do this, but I think the best is to insert one edge, and then - in one step - insert the other one while orienting the top layer edges. You can find some one-step algorithms for this case at Doug Li's speedcubing site.

One possible optimization here is to put in one of the two edges during step 2; this would then allow you to perform step 3 in one look/alg. Doing this would slightly decrease the freedom you have during step 2, though, so it's up to you whether it's worth it or not.

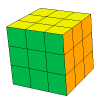
CSO stands for "corner slot + orient". This step is also known as CLS, because it is the same as the CLS step in Lucas Garron's MGLS method. In this step, the goal is to insert the bottom layer corner, while also orienting the top layer corners. It's best to do this in one look, because it can be very fast (and every possible case can be done 2-gen!), but the downside is that it's about 100 algs. You can find them all at Lucas's MGLS page. Personally, I don't like learning algs that much, so I just insert the corner in one step and then orient the top edges in one step - that can be pretty fast too.

An important note: it may actually be faster to do CSO first, especially if your ESO algs don't affect corner orientation. The reason is that many of the long CSO algs can be made shorter without the restriction that they don't affect edge orientation. For instance, r U r' U r U' r'. Nobody's generated algs for this yet, but it's an interesting option.

This is the same as the last step of Fridrich, although you'll want to remember to adjust the D face at some point. There are 21 total cases, almost all of which can be done very fast, so it's certainly worth it to do this in one step. I would say the best page for this step is the one on the SpeedSolving wiki, which has a huge number of algs for each case, so you can find the one that feels most comfortable for you.